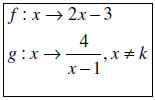Mathematics

EXERCISE 1.2

1. Given function f is defined by f (x) = 5x − 4 . Find the value of x if f (x) = −14 .


2. Given that f (x) = 2x − 3. Find the image of 5.
3. 
   (i) The image of 5.
   (ii) the value of x such that the function f is undefined


4. Given the function h such that
   h(x) = 2x²+3kx+1 and h(1) = 9. Find the value of k.


5. Given the function f : x →3x +1, find
   (a) the image of 2,
   (b) the value of x if f(x) = x.


6. Given the function w : x → 4x + 3, find the value of p such that w(p) = 23 .
7. Given the function n : x → 2x − 5. Find the value of k at which n(k) = k .

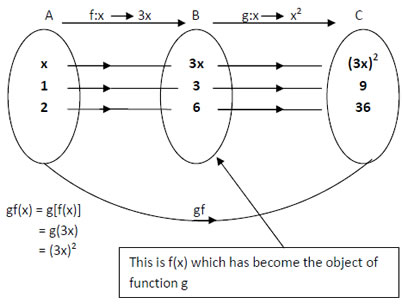
The figure shows Set A, B and C.
The combined effect of two
functions can be represented by
the function gf(x) and not fg(x).
This is because the range of f has
become the domain of function g
or f(x) becomes the domain of
function g.

The concept is:
f(x) = 3x
f(k) = 3(k)
f(3) = 3(3)
f[g(x) ]= 3g(x)

Example 1:

Given function f : x → x +1and g : x → 3x . Find the composite function gf.

Example 2 :

The following information refers to the functions f and g.

Find
(a) the value of k,
(b) fg(x).