- English
- Sociology
- Functional English
- Psychology
- Mathematics
- Philosopy
- Physics
- Computer Science
- Chemistry
- Entrepreneurship
- Biology
- Informatics Practices
- Geography
- Multimedia & Web Technology
- Economics
- Biotechnology
- Business Studies
- Physical Education
- Accountancy
- Fine Arts
- Political Science
- History
- Agriculture
CBSE Guess > Papers > Question Papers > Class XII > 2003 > Mathematics > Outside Delhi Set -I
MATHEMATICS—2003 (Set I — Outside Delhi)
SECTION - A
Q. 1. Evaluate: 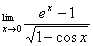
Q. 2. Differentiate 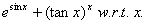
Or
Differentiate 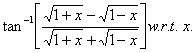
Q. 3. Evaluate: 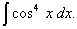
Q. 4. Evaluate: 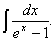
Or
Evaluate: 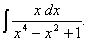
Q. 5. Find the differential equation of the family of curves given by 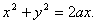
Q. 6. Solve the differential equation, given that 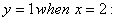
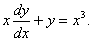
Q. 7.  find k such that
find k such that 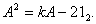
Q. 8. Using the properties of determinants, show that

Q. 9. Find the derivative of sin  from first principles.
from first principles.
Q. 10. Find the intervals on which the function  is
is
(a) Increasing,
(b) Decreasing.
Q. 11. Evaluate: 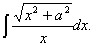
Or
Evaluate: 
Q. 12. Evaluate: 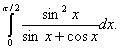
![]()
Or
Evaluate: 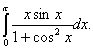
Q. 13. Find the Kari Pearson's coefficient of correlation between  from the following data:
from the following data:
x |
10 |
7 |
12 |
15 |
9 |
15 |
8 |
y |
6 |
4 |
7 |
10 |
11 |
8 |
10 |
Interpret the result also.
Q. 14. A bag contains 8 red, 3 white and 9 blue balls. If three balls are drawn at random, determine the probability that
(a) All the three balls are blue balls.
(b) All the balls are of different colours.
Q. 15. In a single throw of two dice, find the probability that neither a doublet nor a total of 10 will appear:
Q. 16. An open tank with a square base and vertical sides is to be constructed from a metal sheet so as to hold a given quantity of water. Show that the cost of the material will be least when the depth of the tank is half of its width.
Q. 17. Using matrix method solve the following system of linear equations:
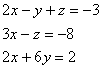
Q. 18. Prove that the area enclosed by  square units.
square units.
SECTION - B
Q. 19. Using vectors prove that 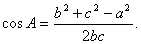
Q. 20. Given that  verify that
verify that 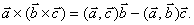
Q. 21. A particle is projected so as to graze the tops of two walls, each of high 10 m at distances 15 m and 45 m respectively from the point of projection. Prove that the angle of projection is tan 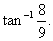
Q. 22. A stone is projected vertically upward with a velocity sufficient to carry it to a height of 50 m. Find its velocity of projection and the time taken to come back to the ground. [Use g = 9.8 m/s2 ]
Q. 23. Slove the differential equation  give that
give that 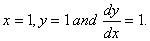
Q. 24. The Cartesian equations of a line are 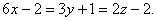
Find (a) the direction ratios of the line, and (b) Cartesian and vector equations of the line parallel to this line and passing through the point (2, -1, -1)
Q. 25. If the plane  touches the sphere,
touches the sphere,  find the value of
find the value of 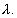
Q. 26. Three forces P, Q and R acting at a point are in equilibrium. The angle between P and Q is double the angle between P and Q. Prove that
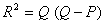
Or
The horizontal roadways of a bridge AB, 12 m long, weight 56000 N and rests on two supports at its ends, What is the pressure on each support when a lorry of weight 33600 N, Starting from A, is at a point two- third of the on the bridge?
SECTION - C
Q. 19. Three bags contain balls as shown in the following table:
Bag |
Number of |
||
White balls |
Black balls |
Red balls |
|
I II III |
1 2 4 |
2 |
3 1 2 |
A bag is chose at random and two balls are drawn. They happen to be white and red. What is the probability that they came from the third bag?
Q. 20. The first four moments of a distribution about x=2 are 1, 2.5, 5,5 and 16 respectively. Calculate the four moments about the mean.
Q. 21. A starts a business with a capital of Rs. 4,00,000. After 3 months B joins him with a capital of Rs. 6,00,000 and at the end of another 3 months C joins with Rs. 10,00,000. If at the end of the year, the total profit be Rs, 5,40,000, and they share it in the ratio of their effective capitals, calculate the shares of A, B and C in the total profit.
Q. 22. The cost function of a firm is given by 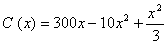
Calculate the output at which the marginal cost is minimum. Also calculate the minimum marginal cost.
Q. 23. Calculate Bowley's coefficient of skewness for the following data:
x |
5 |
10 |
15 |
20 |
25 |
30 |
35 |
40 |
45 |
y |
9 |
8 |
12 |
15 |
11 |
7 |
6 |
5 |
2 |
Q. 24. Find the present value of an ordinary annuity of Rs. 500 per annum for 12 years at 15% per annum. [Given that 1.15) 12 = 5.35]
Q. 25. An insurance company has discovered that only 0.1% of the population is involved in a certain type of accident every year. If its 1000 policy holder are selected at random from the population, what is the probability that not more than 5 of its clients are involved in such accidents next year? [Use e -1 = 0.368]
Q. 26. A house wife to mix up two kinds of foods X and Y in such a way that mixture contains at least 10 units of vitamin A, 12 units of vitamin B and 8 units off vitamin C. The vitamin contents of 1 kg of food X and 1 kg of food Y are as given in the following table
Food |
Vitamin A |
Vitamin B |
Vitamin C |
X |
1 |
2 |
3 |
If one kg of food X costs Rs. 6 and one kg of food Y costs Rs. 10, find the least cost of the mixture which will produce the desired diet.
Or
A manufacture makes two types of cups, A and B. Three machines are required to manufacture the cups and the time in minutes required by each is as given below:
Type of Cup |
Machines |
||
I |
II |
III |
|
A B |
12 6 |
18 |
6 9 |
Each machine is available for a maximum period of 6 hours per day. If the profit on each cup A is 75 paise, and on B it is 50 paise, Show that the 15 cups of type A and 30 cups of type B should be manufactured per day to get the maximum profit.
| Mathematics 2003 Question Papers Class XII | |||||||
| Delhi | Outside Delhi | Compartment Delhi | Compartment Outside Delhi | ||||
| Set I | Set I | Set I | Set I | ||||
| Set II | Set II | Set II | Set II | ||||
| Set III | Set III | ||||||
