Chapter 12 : Troigonometry

Trigonometry Identities

Let us take any acute angle taking point P on OB we draw perpendicular PQ on OAB. Thus we have a right angled triangle OPQ. If we suppose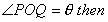

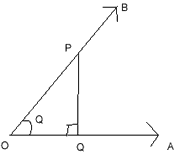
We have OQ2 + PQ2 = OP2--------------(1)

Dividing both sides of (i) by OP2 we get

Similarly, dividing both sides of (1) by OQ2 we get

A gain dividing both sides of (i) by PQ2 , we get

Here, (2), (3) and (4) are all identities. These can be used to express one trigonometric ratio to other trigonometric ratio. For example:

Similarly,

 

Privious Next
Subjects Maths (Part-1) by Mr. M. P. Keshari
Chapter 9 Circle
Chapter 10 Tangents to a circle
Chapter 11 Geometrical Construction
Chapter 12 Troigonometry
Chapter 13 Height and Distance
Chapter 14 Mensuration
Chapter 15 Statistics
Chapter 16 Probability
Chapter 17 Co-ordinate Geometry